● November 30

Kawazumi, Nariya (University of Tokyo)

- Title: Dehn twists and the Goldman-Turaev Lie bialgebra

- Abstract: Using a canonical action of the Goldman Lie algebra on the group ring of a compact oriented surface, we obtain an explicit description of Dehn twist actions on the group ring in terms of the square of the logarithms. This observation induces an embedding of the Torelli group into the (completed) Goldman Lie algebra. The image of the embedding is included in the kernel of the Turaev cobracket. Hence the cobracket gives us a constraint of the Johnson image. Because the formality problem of the Turaev cobracket is solved affirmatively, we find out that the constraint is equivalent to a known one, the Enomoto-Satoh obstraction. This talk is based on some joint works with A. Alekseev, Y. Kuno and F. Naef.

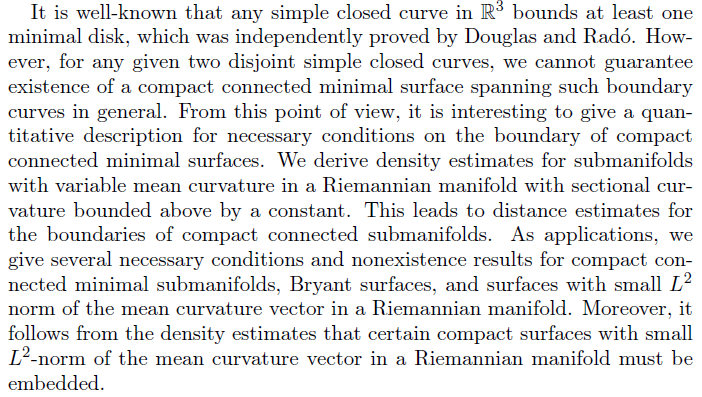
Seo, Keomkyo (Sookmyung Women's Univeristy)

- Title: Connectedness and embeddedness of submanifolds

- Abstract:

Masai, Hidetoshi (Tokyo Institute of Technology)

- Title: Topological entropy of random walks on the mapping class group.

- Abstract: We consider the mapping class group of surfaces. Except for a few sporadic cases, the mapping class groups contain elements called pseudo-Anosovs. The dilatation of pseudo-Anosov mapping classes is a fundamental invariant, and it is characterized in several different ways. One important characterization of the dilatation is that its logarithm agrees with so-called the topological entropy. In this talk, we define topological entropy for random walks and discuss relation to other invariants of random walks. If time permits, we also discuss its dependency of topological entropy on the transition probability measures of random walks.

Scarinci, Carlos (KIAS)

- Title: Ideal tetrahedra and their duals in 3-dimensional spacetimes

- Abstract: In this talk I will present a unified description of hyperbolic, half-pipe and anti-de Sitter ideal tetrahedra based on generalised complex numbers. I will then introduce a new kind of tetrahedra in de Sitter, Minkowski and anti-de Sitter spaces using projective duality, and will discuss some of their properties and relations to generalised shear coordinates on the moduli spaces of constant curvature spacetimes in 3-dimensions.

Ueda, Kazushi (University of Tokyo)

- Title: Reconstruction of K3 surfaces from their Gromov-Hausdorff limits

- Abstract: It is known by Gross-Wilson that a sequence of Calabi-Yau metrics on a general elliptic K3 surface with a fixed diameter converges in the Gromov-Hausdorff topology to a sphere with a Monge-Ampere metric with singularities as the volume of the fiber goes to zero. In the talk, we will discuss the unique reconstruction of a general elliptic K3 surface from the Gromov-Hausdorff limit, and its relation to mirror symmetry. This is a joint work with Kenji Hashimoto.

● December 1

Kim, Inkang (KIAS)

- Title: Plurisubharmonicity of energy function on Teichmuller space

- Abstract: We show that the energy function of harmonic maps from a Riemannian manifold into a Riemann surface is plurisubharmonic on Teichmuller space. We give some applications for energy function along the Weil-Petersson geodesic and  geodesic length functions. This is a joint work with Wan and Zhang.

Irie, Kei (University of Tokyo)

Park, Byungdo (KIAS)

- Title: Cycle maps in differential K-theory

- Abstract: Differential K-theory is a construction on smooth manifolds that combines topological K-theory with differential forms in homotopy-theoretic manner. It has applications in classifying Ramond-Ramond fields in Type II superstring theory, formulating T-duality, as well as the theory of sheaves of infinity category of spectra. So far several models of differential K-theory have been discovered, and each model has its own advantage, but it is in general tricky to prove that two different models are naturally isomorphic. And yet there are analogous homotopy theoretic and algebraic constructions which are similar to differential K-theory but one has to be satisfied with by having a cycle map to the differential K-theory. I will begin my talk with an introduction for a general audience and then introduce outcomes from this research which will include a joint work with Arthur Parzygnat, Corbett Redden, and Augusto Stoffel.

Konno, Hokuto (University of Tokyo)

- Title: Gauge theory for families of 4-manifolds

- Abstract: Gauge theory is one of strong tools to study a 4-manifold. Although a single 4-manifold has been a typical object on which one studies gauge theory, in this talk, I will apply gauge theory for a family of 4-manifolds rather than a single 4-manifold. In particular, I will explain that one can construct characteristic classes of 4-manifold bundles using Seiberg-Witten theory. These characteristic classes are extensions of the Seiberg-Witten invariant to families of 4-manifolds, and can detect non-triviality of smooth 4-manifold bundles.

Cha, Jae Choon (Postech-KIAS)