Hyman Bass (University of Michigan)

Gergely Bérczi (Aarhus University)     [Lecture Note]

Steven Bradlow (University of Illinois at Urbana-Champaign)     [Lecture Note]

- Title: Higgs bundles for isogenous Lie group 
- Abstract: Higgs bundles on a closed Riemann surface are defined for any reductive Lie group and have moduli spaces with many intriguing properties. After introducing the objects under discussion I will discuss some interesting relations among Higgs bundles, especially from the point of view of their spectral data, that result from isogenies among low dimensional Lie groups. 

Michel Brion (Université Grenoble Alpes)

- Title: Automorphism groups of almost homogeneous varieties

- Abstract: The automorphism group of a projective algebraic variety X is known to be a "locally algebraic group", extension of a discrete group (the group of components) by a connected algebraic group. The group of components of Aut(X) is quite mysterious: recently, Lesieutre constructed examples for which this group is not  finitely generated. In this talk, we will discuss the structure of Aut(X) when X has an action of an algebraic group with an open dense orbit. In particular, we will see that the group of components is arithmetic (and hence finitely presented) under this assumption.

Corrado De Concini (Università di Roma)

- Title: Projective Wonderful Models for Toric Arrangements and their Cohomology

- Abstract: Joint wih Giovanni Gaiffi.

I plan to sketch an algorithmic procedure which allows to build projective wonderful models for the complement of a toric arrangement in a n-dimensional algebraic torus T in analogy with the case of subspaces in a linear or projective space. The main step of the construction is a combinatorical algorithm that produces a projective toric variety in which the closure of each layer of the arrangement is smooth.

The explicit procedure of our consturction allows us to describe the integer cohomology rings of such models by generators and relations.

Raymond Hoobler (City University of New York)     [Lecture Note]

- Title: Differential Brauer Group

- Abstract: We introduce the differential Brauer group in the affine case by working in the category of sheaves equipped with a family of derivations. Understanding differential Azumaya algebras cohomologically requires locally solving systems of linear differential equations which is done by introducing the Delta-finite Grothendieck topology. We compare this topology with Zariski and etale topologies for appropriate coefficients and then use it to relate the differential and usual Brauer groups. Finally we look at the situation for smooth, projective varieties.

Sheldon Katz (University of Illinois at Urbana-Champaign)

- Title: Affine Lie algebra representations and moduli spaces of sheaves on surfaces

- Abstract: In this talk, I describe a representation of the affine E8 Lie algebra on the Chow group of the moduli space of 1-dimensional stable sheaves on a rational elliptic surface.  This gives a precise mathematical explanation of the physicists' "affine E8 global symmetry of the half K3 surface".  This construction and proof extends to representations of generalized Kac-Moody Lie algebras on the Chow group of the moduli of stable 1-dimensional sheaves on other surfaces.  This is joint with Davesh Maulik.

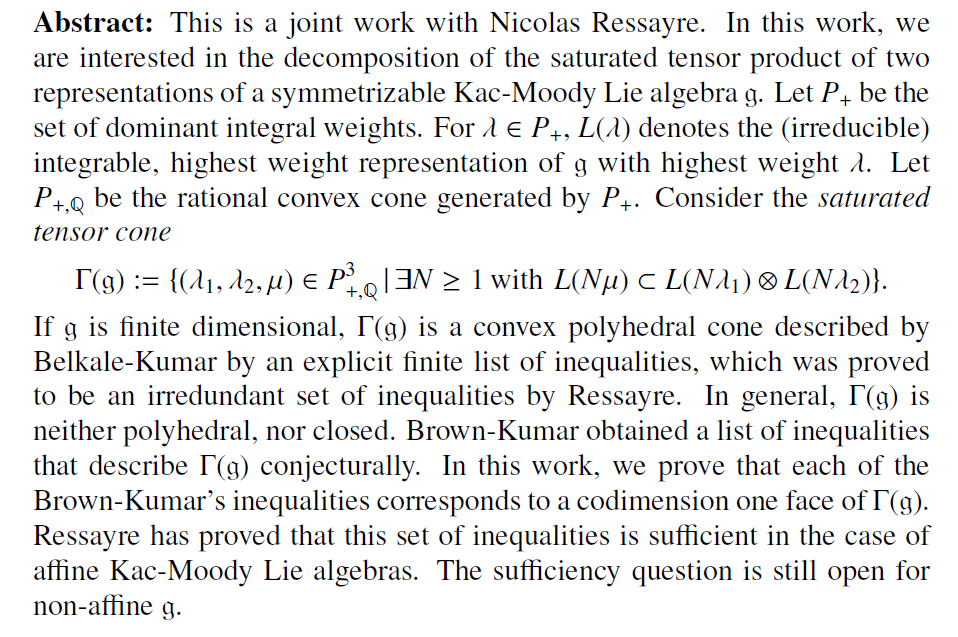
Shrawan Kumar (University of North Carolina at Chapel Hill)

- Title: Facets of tensor cone of symmetrizable Kac-Moody Lie algebras

Niels Lauritzen (Aarhus University)

- Title: Finitely generated bimodules over Weyl algebras.

- Abstract: An endomorphism of a Weyl algebra gives rise to a natural bimodule over the Weyl algebra. Bavula proved holonomicity of this bimodule in characteristic zero. We will sketch recent results (joint with Thomsen) related to this bimodule structure focusing on reduction to positive characteristic and reduction to characteristic zero. The (distant) goal is the illustrious Dixmier conjecture.

Cheolgyu Lee (KIAS)     [Lecture Note]    

- Title: Worst unstable points of a Hilbert scheme

- Abstract: In this talk, we will compute the explicit formula of an arbitrary 0-dimensional projective scheme which is worst unstable with respect to all but finitely many choices of Plucker coordinate. Such schemes have a unique closed point, as we can expect from the case of points on a line.

Masayoshi Miyanishi (Kwansei Gakuin University)

- Title: Triviality of affine space fibrations

- Abstarct: Given an affine space fibration $f : X to Y$, the generic fiber $X_eta:=Xtimes_Y{rm Spec} k(Y)$ is a $k(Y)$-form of the affine space by the generic equivalence theorem of Kraft-Russell. We consider the triviality of $X_eta$ when the fiber dimension is greater than two and the fibration has a relative action of the additive group $G_a$ or the multiplicative group $G_m$. With an additional assumption that a given $G_a$-action is proper and $q$-tight, we give another proof of the existence (Seshadri’s theorem) of geometric quotient by $G_a$ as well as two new proofs of Kaliman's theorem, one proof modulo one of two conjectures on fibrations and the other by studying singular fibers of ${bf A}^1$-fibrations. We also give a theorem on relative linealization of $G_m$-action on ${bf A}^4$.

Brian Parshall (University of Virginia)     [Lectrue Note]

- Title: Some new extensions of Hecke endomorphism algebras II

- Abstract: This talk is joint work with Jie Du (UNSW) and Leonard Scott (UVA), in progress.

As is well-known, the (cross-characteristic) representation theory of the finite groups $GL_n(q)$ can be studied using the famous $q$-Schur algebra, which itself is realized as a Hecke endomorphism algebra. This talk concerns, for the other finite groups of Lie type, an enlargement of the $q$-Schur algebra, based on Kazhdan-Lusztig cell theory, and new ways to use it. In all types the algebra is stratified, and if the characteristic of the field $F_q$ is not a bad prime, it is quasi-hereditary. Interestingly, the proof of the main theorem is based in a non-trivial way on some new constructions of a categorical nature. The talk will be a continuation of Leonard Scott’s talk.

Leonard Scott (University of Virginia)     [Lecture Note]

- Title: Some new extensions of Hecke endomorphism algebras I

- Abstract: This is the first of two talks by myself and Brian Parshall. It is based on our joint work with Jie Du, in progress. I will give some of the history and framework leading to the main conjecture we had made, asserting the existence of a kind of generalized q-Schur algebra, suitable for studying cross-characteristic representation theory of finite groups of Lie type. The conjecture is now a theorem, with some of its proof to be sketched in Parshall’s talk. I will mention some applications as time permits.